1. I. Introduction
owadays many events threaten the Tunisian economy and compel the economists to develop the monetary policy. We note for example the growing public debts illustrated by the change in levels of debt/GDP ratio in major industrialized countries from 2007 to 2010. According to the International Monetary Fund(IMF), the budget deficit in Tunisia evolve from 1.1% in 2010 to 3.5% of GDP in 2011 hence the ratio(debt/GDP) increase from 40% of GDP in 2010 to 44.5% of GDP in 2011.Also the inflation rate was 4.9 %in 2008 and becomes 6% in 2014. (Amiri and Talbi, 2013) underlined the evolution of the inflation in Tunisia .. It was under control after a spike in August 2006 to 4.490% due to the rise in international oil prices, the periods of excess of liquidity and the deteriorating of the exchange terms. However in may 2007, it recognizes an important reduction to 2% due to the monetary tightening and the acceleration of the real GDP' s growth to 5% 1 (CPI) was around 4.43% in 2011 and begins to goes up to 5.15% in 2010..The aim of this study is to highlight the monetary transmission mechanisms (MTM). In this field, (Boughrara, 2007) criticized the limited knowledge of this mechanism in Tunisia considered as an handicap that delays the implementation of Inflation Targeting (IT). This paper also intend to show the main driving forces behind Tunisian business cycle and to understand how the shocks and monetary policy of inflation targeting transmit into the Tunisian economy using the Dynamic Stochastic General Equilibrium model (DSGE) model. In fact DSGE is chosen in this case because it had several attractive advantages for the analysis of the macroeconomic policy compared to VAR, like for example the escapement from the Lucas critique, the fact that they are structural, microfounded. The model is forward looking as it sets the importance of expectations of the agents and the credibility of the Central Bank (Tetlow, 1999). It also provides an integrated approach to the study of the business cycle and the optimal response of the policy-makers to shocks. It evaluates the success of the adoption of inflation targeting regime.However, issues like the technical capabilities and the Central Bank's autonomy, are most of time lacking in the most emerging market economies, so they are not explicitly considered in our context. The Monetary policy of the Central bank in this case is represented by the generalized Taylor rule. We should remember that the subject of inflation targeting became more important since the vast literature on "the Science of Monetary Policy" concentrates on the industrial countries and the developed emerging markets (Clarida et al.1999;Taylor, 1998;Villarverde, 2009;Lubik and Shorfheide, 2007). Even so , there is a few literature about the emergent countries implementing targeting we note for instance (Peiris and Saxegaard, 2007 ;Benes et al., 2007;Ben Aissa and Rebei, 2009). As far as the objectives of the monetary policy are concerned. We note for instance stabilizing the prices' level, the economic growth, the financial market and minimizing the unemployment. In accordance with the Article 33 of the new Law No. 2006-26 concerning the organization of the BCT (Central Bank of Tunisia), the objective of monetary policy is to maintain the prices stability. To this end, the issuing institution will use the interest rate as a basic instrument to act on the prices (Chockri and Frihka,2011). They elucidated that the Tunisian central bank intend to keep the inflation rate to the level comparable to its partners and competitors 2 (especially the European union). They added that the Tunisian monetary authority adjust systematically the exchange rate to maintain the inflation differential, and therefore preserve the external competitiveness. This paper focuses on stabilizing prices via the technique of the inflation target.Since 1987, the Tunisian monetary authority tried to ensure a policy of economic growth and to control the inflationary pressure. So it created a financial sector reform in 1988 via the liberalization of the interest rates and the gradual removal of the volume 's control and the composition of the credits. In fact, the monetary policy in Tunisia recognized a deep mutation. It replaces the direct and discretionary instruments by the intervention in the monetary market. Also it adopted a device which consist in targeting the currency (M3) whose operational target is the monetary base. In 2010,the Board of Directors of the Central Bank decided the adoption of a monetary policy which aimed at controlling the inflation , ensuring an adequate financement of the economy to maintain unchanged the interest rate .It also monitors the shift in the international conjunctures and its possible impact on the national economy and the balance of payments in particular (Amiri andTalbi,2013).The inflation targeting monetary strategy is not a new approach. Indeed, it was first implemented in New Zealand in 1990, then in the developed economies like Finland, Sweden, Australia, The Czech Republic, Poland, Brazil, Chile, Colombia, South Africa, Thailand, South Korea, Mexico etc followed this strategy. The success that New Zealand, Canada or Great Britain is witnessed through an inflation that does not overcome the claimed in flation target (Ammer and Freeman, 1995). Inflation targeting was adopted only in1999 by the developed countries (Leiderman and Svenson, 1995;McCalum, 1996;Bernanke et al. 1999). Given the benefits of this policy in terms of credibility and anchor expectations, Tunisia plans to adopt it in the medium run, also it is guided to a flexible exchange rate. According to (Baccar, 2008), the Tunisian governor of the Central Bank at that time, IT is closely linked to the capital account liberalization, the total convertibility of the currencies, the flexibility of the exchange rate, the competitiveness of the country, the unemployment, the independency of the Central Bank, the depth of the financial market and the banking system's strength.
The paper is outlined as follows. Section 2 discusses the modeling strategy of the DSGE model. Section3 briefly outlines the estimation procedure and describes the estimation results using the Bayesian technique (Smets and Wouters, 2002) to find the posterior mode, the variance decomposition and the impulse response function of the macroeconomic variables to different shocks. Section 4 summarizes the main findings of this paper.
2. II. Dsge Model for the Monetary Policy Analyses
Actually much research has been conducted to investigate the impact of (IT )on the macroeconomic variables . For example (Vas?cek and Musil, 2005) analyzed the behavior of the Czech economy with (IT) regime following DSGE model. They found that the model seems to give a very satisfactory approximation of the Czech economy behavior. (Benes et al. 2007) used a DSGE model to support the monetary policy in the emerging market economies following ( IT). They tested the model's properties on recent Turkish data, and show that the main stylized features relevant for the monetary policy making, are well captured by the model. (Vîtola and Ajevskis, 2011) assessed the implications of IT versus the fixed exchange rate regime for seven EU non-euro area countries with DSGE model. They found that in all the countries the IT monetary rule guarantees more stable inflation and output than under the other regimes i.e fixed or flexible exchange rate. (Burriel et al,2010) described the main features of the Spanish economy for the policy analysis using the MEDEA (Modelo de Equilibrio Dinámico de laEconomía Española) type of DSGE model which is estimated with the Bayesian techniques, and performed different exercises to illustrate the potential of MEDEA. (Peiris and Saxeegard, 2007) evaluated the monetary policytradeoffs like the exchange rate peg and the IT in lowincome countries using a (DSGE) model estimated on Mozambique data taking into account the sources of major exogenous shocks, and the level of the financial development. The transition to the IT regime needs the comprehension of the monetary transmission mechanism. To do so, the basic New-Keynesian model (the DSGE) in the spirit of (Villaverde, 2009) is described. In this model 3 agents are presented. Begining by the households, the latter represent a continuum so that we can obtain a whole distribution of wages with each household charging its own differentiated wage. The need of a balanced growth path implies that the household maximizes the lifetime utility of the form:
The utility function depends on the consumption c t , employment l t and real money balances mb t /p t. In this case E 0 is the conditional expectation operator, p t is the price level,?? is the intertemporal discount factor representing time preferences. The period utility function is affected by two shocks ? d and ? ? which are normally distributed N(0,1). . The demand shock is an intertemporal preference shock d t which makes the agent consume more or less in the current period.
The other shock is a shock to labor supply ?? t that captures the movements in the observed wedge relating to the preference between leisure and labor(Hall, 1997).
Both shocks follow an autoregressive process of order 1.Their autoregressive coefficient and their standard deviation are respectively ? d , ? ? and ? d , ? ?. The household can save on capital k t-1 with a depreciation rate ?, so that the investment on physical capital x t satisfies : They can also save on money balances mb t , or government bonds b t paying a nominal gross interest rate of R t. The first order condition (FOC) of the household are: Where ? t is the lagrangian multiplier associated with the budget constraint.
The equation above is the leisure choice where ?? is the frish labor supply elasticity and ? the weight on leisure. The pricing kernel is defined by: The final goods' producing firm produces the final domestic goods (y t ) using the intermediate domestic goods (y it ) with the following production function:
where ? is the elasticity of substitution between consumption today and tomorrow across the domestic intermediate goods. The final goods' firms maximize profits in a perfectly competitive environment subject to the production function.
As far as the Intermediate goods' producers are concerned ,there is a continuum of intermediate goods' producers in a competitive market who rent the capital and labor .Each one is defined by i producer and has access to technology represented by a production function:
Where ? is the elasticity of output to capital, A is a scaling parameter which stands for a neutral technology level,k it-1 is the capital rented by the firm, l it is the amount of labour input rented by the firm and z t is the technology growth rate which follows AR (1) process which is defined by: It is worth stating that the firm has constant returns to the scale, so the output rises by the same proportional change in inputs. The marginal cost does not depend on i, i.e all the firms receive the same technology shocks and all the firms rent inputs at the same price. The marginal cost is given by: Where r t is the rental price of capital.The intermediate goods' producers consider that they are subject to a Calvo pricing mechanism, so they choose the price that maximizes the discounted real profits. In each period a fraction 1-? is considered of the firms that can change their prices. It is the probability of reoptimizing prices, while the others keep the previous prices. The firm change their prices to satisfy:
The recursive equation of prices:
The recursive equation for prices 2 :
The recursive condition for prices 3:
g t 2 = ? t ? t * y t + ?? E t ? t+1 ??1 ? ? t * ? t+1 * ? g t+1 2(15)g t 1 = ? t mc t y t +?? E t ? t+1 ? g t+1 1 (14)?g t 1 = (? ? 1)g t 2 (13) p t 1-? =? p t-1 1-? +(1-?) p* t 1-? (12)mc t = ? 1 1 ? ? ? 1?? ? 1 ? ? ? w t 1?? r t ? Ae (1??)z t (11) z t =? z z t-1 +? z ? z,t where ? z,t ~N(0,1)(10)y it= Ak it ?1 ? (e z t l it ) 1?? (9) y t = ?? y it ??1 ? 1 0 di? ??1 ? (8) m t = ? ? t ? t?1(7)e ? t ?l t ? c t =w t (6)e d t c t =? t(5)k t =(1-?)k t-1 +x t ,(4)? t =? ? ? t-1 +? ? ? ?,t(3)d t =? d d t-1 +? d ? d,t(2)E 0 ? ? t e d t ? t=0 ? log c t ? e ? t ? l t 1+? 1+? + v log ( m b t p t )?(1)The government is considered as the last agent in the model. The latter decides the nominal interest rates through the open market operations that are financed through lump-sum transfers T t according to the following Taylor rule as a function of the past interest rates, and the inflation :
In this Taylor rule ? is the target level of inflation(equal to the inflation in the steady state)and R= ? ?? is the steady state nominal gross return of capital. ? mt is a random shock to the monetary policy normally distributed with an autoregressive coefficient ? m and a standard deviation ? m . The implementation of the interest rate can be shown either through the open market operations or through the paying interest on bank reserves. The monetary policy yields either a surplus or a deficit in both cases that is eliminated through lump-sum transfers T t to the households. The equilibrium of the economy is characterized by the first order conditions of the household, the firms, the taylor rule of the government and the market clearing.
3. III. The Bayesian Estimation of the dsge Model
To ensure that the model is not stochastically singular and to reproduce the dynamic in the data, a number of stochastic shocks are incorporated in the model. The shocks that affect the economy in this case are the intertemporal, the productivity,the labor supply and the monetary shock. To estimate the parameters of the DSGE model, the data are quarterly chosen covering the time interval from 1980Q1 to 2011Q4.We use time series for 7 macro-economic variables in Tunisia which are defined by the real gross domestic product(GDP), the consumption, the total investment, the inflation, the real wages, the employment and the nominal interest rate. All the data are supplied by the International Financial Statictics (IFS),the web site of the Central Bank of Tunisia, and the National Statistics Institute of Tunisia web site(INS). The output, consumption, investment, employment were transformed to quarterly data using low to high quadratic-much -sum converter of EViews. The macroeconomic variables were transformed into real per capita. The missing data are filled by K Nearest Neighbor (KNN ) of matlab. According to (Juillard et al. 2004) the time trend in the data are removed on the key macro variables with Hodrick Prescott filter. All the seasonal features of the series are removed using X12 ARIMA FILTER because the theoretical model is not designed to pick up the seasonal fluctuations in the macro -economic data. The seasonally adjusted data are also considered in logarithms except the wage rate. The DSGE model is estimated using the Bayesian techniques. It is worth reminding that this approach which characterizes the posterior distribution of the parameters of the model places a prior distribution p(?) on the deep parameters and updates the prior through the likelihood function. (Geweke, 1998) shows that this is directly related to the predictive performance of the model and it is useful for the policy analysis and forecast. This Bayesian approach enables us to process the model and the uncertainty parameter explicitly and seeks a model with the highest posterior probability. For the calibrated values which are quite standard in the literature, the paper refers to the values of (Villaverde, 2009) and (Ben Aissa and Rebei, 2009) which are based on the parameterization of (Backus and Crucini, 2000).The quarterly discount factor ? in equation( 1)is set equal to 0.98 so that a yearly nominal R interest rate is about 4% at the steady-state. The latter is annualized so that ? = exp[-r/400].The steady state rate of inflation ?? defined in the equation ( 16) is set to 1.005.
The quarterly depreciation rate of capital ??=0.025 noted in the equation( 4)is chosen like the value calibrated in the low income countries detected by (Peiris and Saxegaard, 2007). The persistence of all the shock ? ? ,? z, ? d presented respectively in equation( 3),( 10) and ( 2)is set to 0.8 but their associated standard deviation are different. For example, the standard deviation for the productivity shock determined in equation (10) in our case is very low ? z = 0.01 according to (Gali and Monacelli, 2005) and to the average of the international business cycle literature. This is because the new technologies are usually introduced slowly in respect to the quarterly periods. The standard deviation of the monetary shock expressed in equation( 16) is very low where ? m =0.005 and the corresponding autoregressive coefficient is set to 0 , ? m = 0. Table 1 exhibits the calibrated parameters that are selected according to the literature and by the theoretical restrictions imposed on some of the parameters while the table 2 displays the steady state result . As for the choice of the prior distribution of the parameters. The Beta distribution is selected for the parameters that lie between 0 and 1 such as the autocorrelation coefficients of the shock processes ?. The Normal distribution was chosen or the unconstrained parameters while the Inverse Gamma and gamma distribution was selected for the shocks and the parameter values greater than zero i.e real positive value. For the choice of the mean and the standard deviation of the priors, we refer to the model of (Villaverde, 2009) and (Smets and Wouters, 2007). The average duration in which a firm can optimize its price are centered around four quarters. According to (Smets and Wouters, 2002),it is two and a half years (2,5) .The estimation result are demonstrated in table 3 and 4 inference on the parameters. According to the multivariate and univariate diagnostics, a sample of 100000 draws were sufficient to ensure the convergence of the Metropolis-Hastings sampling algorithm. Especially The MCMC is run with 100000 draws with 2 parallel chains of MH algorithm. In each case of the three figures, dynare reports both within and between the chains' measures. The red line measures the parameter vector within the chains while the blue line measures the parameter vector between the chains. The convergence of the MH method measures computed within and between the chains should converge. An overall convergence is reached after 100000 draws. There are many convergence diagnoses, which enable the determination of the chain convergence. Without convergence veri fication, the approximation of the posterior distribution is not credible (Grabek et al. 2011). Table 3 reports the mode and the mean as well as 90% per cent con fidence interval of the posterior probability which reflects the uncertainty on the structural parameters. The confidence interval reports the 5, and 95 percentile of the standard deviation of the posterior distribution of the parameters obtained through the MH sampling algorithm. The Calvo parameter or the price stickiness parameter of the intermediate good producers for the price adjustment determined in equation ( 12) presents information about the timing of the price and the wage setting decisions by the domestic firms and the households. The posterior mean of this parameter is found ?= 0.4997 i.e 49% of firms do not reoptimize their price setting implying a price duration of the non optimizing firms of 1/(1?)= 1.99 quarters. This means that the nominal prices are fixed for 2 quarters approximatively. This parameter is important but it is low compared to the one of the euro area which is nearly 3 quarters for ?=0.9 see (Smets and Wouters, 2003;Adolfson et al. 2005;Gal? and Gerlter, 1999). Knowing that the inflation is negatively correlated to the price stickiness. If there is a high rigidity, there will be a sluggish or gradual response (increase or decrease)of inflation to a monetary shock (Mankiw, 2000; Christiano et al. 2011). This rigidity rationalizes the persistent effect of the monetary shock and brings about a lesser fluctuation of the variable. The posterior mean of the Frish labor supply elasticity ?? defined in ( 6) is 1.5128. According to (Burriel et al. 2010),the posterior mean of this elasticity in the Spanish economy accounts for 1.83, which is in line with most estimations for the other countries . As an example, (Smets and Wouters, 2007) estimate a high Frisch elasticity of 1.9 . The value found is rather credible once we think about both the intensive and the extensive margin of the labor supply. Also (Hall, 2009) affirmed that the fluctuation in the total hours worked in the economy as the workers involuntarily lose jobs in economic downturns and regain jobs as economic conditions improve, is largely due to the changes in the number of workers who move in and out of unemployment rather than to changes in workers' desired work hours. The elasticity of output to the capital which is the relative shares of the capital in the goods production noticed in equation( 9)is set to ?= 0.4102.According to (Adjemian and Devulder, 2010) this parameter ? obtained from the Cobb-Douglas function is situated between[0,1] interval.They think that it is nearly in the neighborhood of 1/3 as the Year 2015
4. Global Journal of Human Social Science
5. ( E )
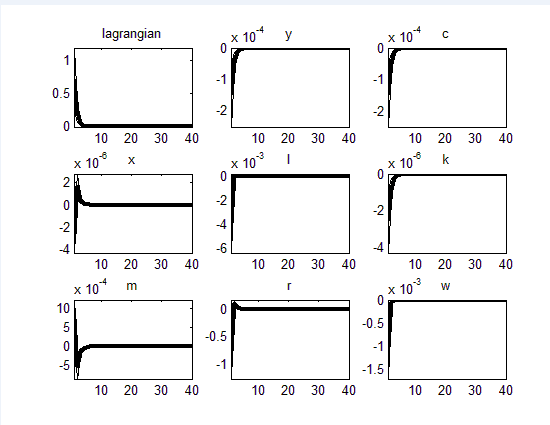
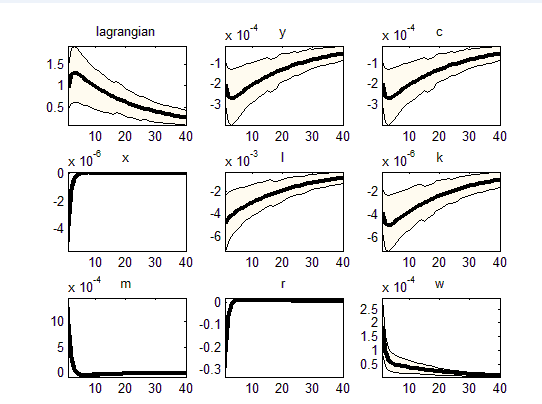
conventional settings. In terms of the posterior mean of the elasticity of substitution of consumption given by equation ( 8) ? ,it amounts to 2.7209.This value is not consistent with the log preferences and the findings of (Casares, 2001) for the euro area and the RBC literature which assumes an elasticity of substitution between a half and one. However, in this case it is highly conspicuous that consumers are less responsive than expected to change their current consumption decisions in response to the interest rate shocks. This value is not very far compared to the inverse of the intertemporal elasticity of the consumption substitution perceived by (Gelain and Kulikov, 2009)which is centered around 1.85.It is higher than the value 1.39 reported by (Smets and Wouters, 2003) for the euro area.When it comes to the estimates of the autoregressive coefficients of the stochastic processes, we can see that they are high as they reflect a high degree of persistence found in the data. Based on the posterior mean, the estimates of the smoothing degree of the preference shock noted in equation( 2) is ? d= 0.6855 considered important.So it has an effect on the permanent change in the preference. The other estimated coefficients imply a higher persistence for the labor supply shock ? ? = 0.9554 detected in equation( 3).This persistence, coming from the labor disturbance, is sufficient to describe the observed dynamics of the Tunisian time series. The persistence for the productivity shock noticed in (10), is ? z = 0.9999 which seems to be in line with the literature as well as with the benchmark studies for the euro area (Smets and Wouters, 2003) and (Adolfson et al, 2005). In this context (Burriel et al. 2010) observed that the estimation of the autoregressive parameters 's shock processes show that the domestic shocks are very persistent, especially those related to demand, public consumption, and preferences.The next set of the parameters reported in Table 4 is related to the standard deviation of the shock. Beginning with the labor supply shock ? ? defined in equation( 3) ,it is estimated to 0.0574 so they are not very important in explaining the business cycle fluctuation. Henceforth, the deviation of the preference shock observed in ( 2) is ? d =0.0566. The monetary shock is larger with a mean of ? m =0.0725. Finally the productivity shock presented in (10) has the value of ? z = 0.5357 which is the most volatile. It is crucial to refer to the previous studies in the Tunisian economy with DSGE. For the instance ,the research carried out by (Ben Aissa and Rebei, 2009) who detected the standard deviation of the technology shock both for the subsidized and the non subsidized intermediate goods which amounts to 0.00925 on average .It is more volatile than the monetary shock set to 0.001 on average, and that of (Jouini and Rebei, 2013) In fact, in the long run, the reduction in the money supply via the interest rate reduces proportionally the inflation. As stated by Friedman "inflation has a monetary origin". Therefore, the currency has no effect on the production and the nominal interest rate in the long run. In absence of the money effect in the long run, we can conclude that it is neutral. 4 plots the intertemporal preference shock or the discount factor shock. The latter is a demand shock which make the agent prefer to consume more or less in the current period. The consumption choices can be affected by the deep habit that it not mentioned in this case. Our result is different from the one of (Smets and Wouters, 2002) focusing on the effect of a positive preference shock, that is an increase in the interest in consumption, and to the temporary shock of (Vasicek and Musil, 2005) in terms of inflation, output and interest rates. It is also different from (Grabek et al. 2011) with thepositive preference shock, in terms of consumption, output, employment ,domestic prices and interest rate. On the other hand, we find that labor supply (l) as well as the real wage(w) reveal a downward trend. This is justified by the decreasing demand in consumption that puts pressure on the real wage which will drop making the marginal cost (mc) defined in (11)and the inflation(ppi) fall. (Smets and Wouters, 2004) claimed that the downturn in the US economy is mostly driven by a negative preference and an investment shock.
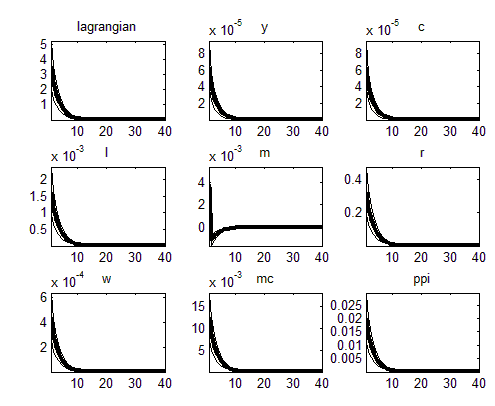
Year 2015 ( E ) The technology or the productivity shock is represented in Figure 5. When there is a high productivity, the representative intermediate goodsproducing firms are able to produce more goods at a lower price. Therefore we can deduce like (Adnen and Frikha, 2011) and (Daboussi et al. 2013), that in the short term, a supply shock has a positive effect on production which is rising (around 10 -2 ). According to the conventional theory, a rise in the productivity and an improvement in the production technology leads to an increase in the output(y) and the consumption(c). In this context,(Jouini and Rebei, 2013) highlighted that the increasing production shock in the goods' sector raises demand throughout the Tunisian economy and hikes the aggregate outputs as well. For more information, we can refer to the results of (Gelain and Kulikov, 2009;Mkrtchyan et al. 2009;Smets and Wouters, 2002;Huseynov, 2010). This shock makes the price level or the inflation(ppi) decline in the short term because the monetary policy cannot offset the decline in the marginal cost (Peiris and Saxegaard, 2007). According to (Mkrtchyan et al. 2009), the inflation decreases owing to the reduction of the production cost and the higher labor productivity. Also (Huseynov, 2010) ascertains that more production and more distribution networks imply a decrease in the domestic price which leads to a drop in the domestic inflation. The interest rate(r) dips in the short run. (Mkrtchyan et al. 2009) and (Benes et al. 2005) claimed that the monetary authority responds to deflation by lowering interest rates. Furtermore, (Grabek et al. 2011) added that lower inflation implies a reduction of the interest rate. This result is similar to the one of (Smets and Wouters, 2003;Gelain and Kulikov,2009). In the medium term, it is important to remember the finding of (Chockri and Frikha, 2011; Daboussi et al. 2013) who revealed that this positive effect of the supply shock on output(y), the nominal interest rate (R n ) and the price level are more likely to decline and vanish. Therefore this shock effect becomes negligible and tends to be absorbed in the long run. Concerning the investment(x), it declines as the result found by (Jouini and Rebei, 2013) in Tunisia because of the depreciation of the real exchange rate according to them. Employment(l) declines as a result of the interaction between the sticky prices and the technological shift pointed out by (Gali,1999;Smets and Wouters,2002;Peiris and Saxegaard, 2007 ;Grabek et al. 2011). (Peiris and Saxegaard, 2007) explained that the reason that makes the firms reduce employment is the decrease of the aggregate price level and the rise of the aggregate demand less than the growth of productivity. (Peiris and Saxegaard, 2007) and (Grabek et al. 2011), discovered that the marginal cost (mc)of the domestic production falls across all the firms due to the rise in productivity (Smets and wouters, 2002).The latter enables them to lower the prices of the domestic goods(Mkrtchyan et al. 2009). Finally, following this positive productivity shock ,the real wage(w) gradually rises in the medium term which is consistent with the result of (Gelain and Kulikov, 2009) and (Smets and Wouters, 2002). Eventually, there is another interesting shock which is the labor-shock interpreted by a rise in the labor supply. This shock is displayed in the figure 6 . Since the end-nineties, there have been significant shifts in the Tunisian economy, mainly due to the increase in the labor force participation, the large immigration flows and the integration of the women and the young manpower in the labor market. It is noticeable that the nominal interest rate(R n ) has increased then leveled off. There is also a wagereduction (w). In this context, (Gelain and Kulikov, 2009) puts forward that when working-hours increase, wages drop which imply a reduction in the domestic inflation(ppi) in the long run via the effect of the reduction of the marginal cost(mc). Indeed, (Smets and Wouters, 2002) showed that the fall in real wages leads to a fall in the marginal cost as well as a decrease in the inflation. To further elucidate the trend of these two variables, one can refer to (Grabek et al. 2011) who confirms that the marginal costs of domestic production as well as the inflation rise in the short term' then will slightly decrease in the medium term. He also justified that an increase in the price of labour services makes the inflation important. The Dynamic Stochastic General Equilibrium Model for the Monetary Policy Analysis in Tunisia
6. Global Journal of Human Social Science
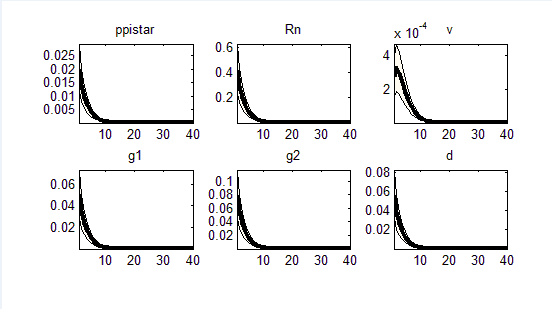
In our case, the output as well as the consumption insignificantly drop in the beginning of the period but will recover very shortly. This reduction in the output is explained by a large negative demand shock, when the labor supply was negative (Burriel et al. 2010). However, in the medium and the long run there is a stimulation of consumption (c) which in turn boosts the output(y) and the investment(x) because of the fall of the inflation (Gelain and Kulikov, 2009). The Employment(l) rises in line with the rise in the output(y) like the findings of (Smets and Wouters, 2002) due to the fall in the leisure. The investment is improved due to the augmentation of the consumption and the reduction of the leisure. The result related to output, consumption and investment the one of (Grabek et al. 2011) in analyzing the increase in the importance of the leisure. According to them, an improvement of the leisure ,leads to the reduction in the investment, the GDP , the employment and the consumption.
7. b) Variance decomposition
The importance of the structural shock which contributes to the dynamics of the state variable in the DSGE model is measured by the share of the total variation that a shock explains for each variable of the model (Gelain and Kulikov, 2009).Table 5 presents the variance decomposition result for the Tunisian economy. The most important shock is the monetary shock ? m which accounts for 50% of the state variables. The second shock is the intertemporal shock ? d defined in (2). It accounts for approximatively 45% of the variance of the variables. The other shocks have a marginal contribution to the overall variability of the model notably the labor shock. Concerning the output variation, like the results of (Smets and Wouters, 2002), they are driven primarily by the monetary shock ? m= 55.81% and the intertempral shock ? d =20.04 %, but the labour and the productivity shocks contribute successively with 4.96 % and 19.20 % of the error variance. Moreover, it is noted that the most striking shock that contributes to the inflation variability is the monetary shock with ? m =74.35%. As (Daboussi et al. 2013), we find the prevalence of the monetary shock over the inflation rate. So an anti-inflation policy is deemed necessary. This is contrary to the result of (Smets and Wouters, 2002) who noticed that the monetary shock account little for the variation of the inflation. Besides, there is the productivity shock with ? z =12.51%. The contribution of the preference shock ? d is not negligible with 10.97 %. In some research previously carried out, there are other shocks like the wage mark up and the price mark up shock that are very crucial in the contribution of the inflation variability.Unfortunately they are not available in this paper.For the interest rate, the most visible shock is also the monetary shock which contributes with ? m =77.16% to the variation of the latter. Then, follows the productivity shock with ? z =10.58 % and the preference shock with the contribution of ? d 10.34 % of the variability of the interest rate.For the employment, the most striking shock is again the monetary shock ? m with 64.22%; then occurs the preference ? d with 22.30% and finally the labor shock that accounts for ? ? = 5.50% of the employment variability. To conclude for the real wage variable, it is necessary to mention that in the study of (Smets and Wouters,2002) the wage mark up shock is the most conspicuous in the variation of the real wage, but in this case, the monetary shock occurs at the top with a part of ? m= 79.77% and the productivity ? z shock comes after with a contribution of 14.49 % of the real wage variability.
8. IV. Conclusion
This paper provides a simple neokeynesian model of (Villaverde, 2009) represented by DSGE to evaluate the transmission of the monetary policy in Tunisia and to see the effect of applying the IT policies. To do so, the Central Bank must stabilize the prices according to the Taylor rule to adjust the interest rate. This model is estimated with the Bayesian techniques with the nominal rigidities using seven macroeconomic variables and 4 shocks. The empirical properties of the model is examined by studying its impulse-response functions and its variance decomposition. The estimated DSGE turned out to have economically plausible properties in terms of the propagation of the key economic shocks into the Tunisian economy and their contribution to the business cycle fluctuations. It seems to show a suitable approximation of the behavior of the Tunisian economy with respect to the results. The findings reveal that the monetary shock bears a significant influence on inflation. The graphics and estimations show the importance of the interest rate channel to stimulate the output and the consumption.. In addition, it is worth noticing that there are some conditions to be met before choosing this regime policy. According to (Mishkin, 2000;Batini and Laxton, 2005), these conditions are the independency of the Central Bank, the advanced infrastructure techniques for a reliable forecasting, the solid financial system, also having a low inflation because of the difficulty of forecasting inflation and hitting an inflation target in the conditions of high and volatile inflation (Hammond, 2012). (Pétursson, 2000) pointed out that there are other factors beyond the control of the Central Bank that have short-term effects on inflation making IT difficult to implement like the fiscal dominance and the labor market conditions. Concerning the limits of this study, (Kvasnicka, 2001) and (Mizen,1998) affirmed that the examples of success achieved by many countries do not mean that IT is the best conduction driven by the monetary policy. Until now researchers don't know whether this regime is more suitable for the developed countries (Masson et al. 1997) or for the emerging countries. They cannot take general conclusions for this policy because the period of implementation it is too short (Jonas and Mishkin, 2003). (Hammond, 2012) added that IT is not the optimal monetary policy regime for curbing inflation but it proved to be effective at anchoring inflation expectation around the target and maintaining inflation stable. Many other features are tremendously essential to capture the persistence and the covariance in the data, to ameliorate the fit of the model and to delay the response of the economy to shocks, are missed in this paper.For example the habit persistence in consumption, the adjustment cost of investment and changing the utilization rate ,the labor market frictions that will enrich the study. In this paper, we put forward a closed small model of the Tunisian economy. In the further research, the open economy model as well as IT with a floating exchange rate as a new monetary policy framework of choice will take our interest , because of the failure of money targeting in the mid1980 and the collapse of the fixed exchange rate pegs in the early 1990 (Hammond, 2012).




| Table1 : Calibrated parameters | |||||||||||
| Parameters | Value | Description | Parameters | value | Description | ||||||
| ? | 0.98 | Discount factor | Autoregressive coefficient | ||||||||
| (persistence) | of | the | |||||||||
| shocks | |||||||||||
| ? | 0.35 | Elasticity of output to | ? d | 0.8 | Degree of smoothing the | ||||||
| capital ratio | preference shock | ||||||||||
| ? | 1/3 | The weight on leisure | ? z | 0.8 | Degree of smoothing the | ||||||
| productivity process | |||||||||||
| ? | 0.5 | Frish | labor | supply | ? ? | 0.8 | Degree of smoothing of labor | ||||
| elasticity | supply shock | ||||||||||
| A | 1 | Technology level | Shock standard deviation | ||||||||
| ? | 0.025 Depreciation | rate | of | ? d | 0.025 | Standard | deviation | of | |||
| capital | preference process | ||||||||||
| ? | 8 | Elasticity of substitution | ? ? | 0.01 | The standard deviation of | ||||||
| labor supply shock | |||||||||||
| ? | 0.75 | a fraction of firms that | ? z | 0.01 | The standard deviation of | ||||||
| keep | the | previous | technology | ||||||||
| price.(does not change | (productivity)shock | ||||||||||
| price) | |||||||||||
| ? | 1.005 Target level of inflation | ? m | 0.005 | The standard deviation to | |||||||
| monetary shock | |||||||||||
| ? | 1.5 | The degree of interest | |||||||||
| rate smoothing | |||||||||||
| Parameter | Value | Description | Parameter | Value | Description | |
| Utility | mc | 0.875037 | Marginal cost | |||
| lagrangian | 1.20317 | Lagrangian | ? | 1.005 | Inflation rate | |
| multiplier | ||||||
| allocation | ? * | 1.01623 | Inflation at the equilibrium | |||
| y | 1 | output | Rn | 1.02551 | Nominal interest rate | |
| according to taylor rule | ||||||
| c | 0.831141 | consumption | v | 1.00144 | Price | dispersion |
| inefficiency | ||||||
| x | 0.168859 | Investment | Auxiliary function | |||
| l | 0.333333 | labour | g1 | 4.47853 | Recursive equation for | |
| prices2 | ||||||
| k | 6.75437 | capital | g2 | 5.11832 | Recursive equation for | |
| prices 3 | ||||||
| Prices | Stochastic | |||||
| process | ||||||
| m | 0.98 | Pricing kernel | d | 0 | Intertemporal shock | |
| r | 0.0454082 | Interest rate | ? | 0 | Labor shock | |
| w | 1.70878 | Real wage | z | 0 | Productivity shock | |
| Parameter | Prior distribution | Prior | Prior | Maximized posterior (estimated maximum | |||
| Mean | Standard | posterior) | |||||
| deviation | mode | mean | Confidence interval | ||||
| Posterior distribution | |||||||
| (MH) | |||||||
| 5% | 95% | ||||||
| ? ( bbeta) | gamma_pdf, | 0.250 | 0.1000 | 0.0705 | 0.0705 | 0.0698 | 0.0712 |
| G2(µ, ?, p3) | |||||||
| ? (ppsi) | normal_pdf, | 9.000 | 3.0000 | 19.9816 | 20.2257 | 20.0599 | 20.4362 |
| N(µ, ?) | |||||||
| ?(zzeta) | normal_pdf, | 1.000 | 0.2500 | 1.5330 | 1.5128 | 1.4888 | 1.5321 |
| N(µ, ?) | |||||||
| ? (aalpha) | normal_pdf, | 0.300 | 0.0250 | 0.4121 | 0.4102 | 0.4079 | 0.4119 |
| N(µ, ?) | |||||||
| ? (ddelta) | beta_pdf, | 0.025 | 0.0030 | 0.0365 | 0.0366 | 0.0363 | 0.0369 |
| B(µ, ?, p3, p4) | |||||||
| ? (eepsilon) | normal_pdf, | 1.500 | 0.3700 | 2.7001 | 2.7209 | 2.6673 | 2.7799 |
| N(µ, ?) | |||||||
| ? (ttheta) | beta_pdf | 0.500 | 0.1000 | 0.4897 | 0.4997 | 0.4911 | 0.5071 |
| B(µ, ?, p3, p4) | |||||||
| ? d (rrhod) | beta_pdf, | 0.500 | 0.2000 | 0.6731 | 0.6855 | 0.6709 | 0.7011 |
| B(µ, ?, p3, p4) | |||||||
| ? ? (rrhophi) | beta_pdf, | 0.500 | 0.2000 | 0.9925 | 0.9554 | 0.9401 | 0.9718 |
| B(µ, ?, p3, p4) | |||||||
| ? z (rrhoz) | beta_pdf | 0.500 | 0.2000 | 0.999 | 0.9999 | 0.9999 | 0.9999 |
| B(µ, ?, p3, p4) | |||||||
| Parameter | domain Prior distribution | Prior | Standard | Maximized posterior | ||||
| Mean | deviation | mode | mean | Confidence interval | ||||
| 5% | 95% | |||||||
| ? d (ssigmad) | R+ | inv_gamma_pdf, | 0.100 | 2.0000 | 0.1302 | 0.0566 | 0.0302 | 0.0832 |
| ? ? (ssigmaphi) | R+ | inv_gamma_pdf | 0.100 | 2.0000 | 0.0396 | 0.0574 | 0.0259 | 0.0828 |
| ? z (ssigmaz) | R+ | inv_gamma_pdf | 0.100 | 2.0000 | 0.5410 | 0.5357 | 0.4801 | 0.5880 |
| ? m (ssigmam) | R+ | inv_gamma_pdf | 0.100 | 2.0000 | 0.0464 | 0.0725 | 0.0245 | 0.1231 |